This course introduces statistical inference, sampling distributions, and confidence intervals. Students will learn how to define and construct good estimators, method of moments estimation, maximum likelihood estimation, and methods of constructing confidence intervals that will extend to more general settings.



Statistical Estimation for Data Science and AI
This course is part of multiple programs.

Instructor: Jem Corcoran
8,801 already enrolled
Included with 
(88 reviews)
Recommended experience
What you'll learn
Identify characteristics of “good” estimators and be able to compare competing estimators.
Construct sound estimators using the techniques of maximum likelihood and method of moments estimation.
Construct and interpret confidence intervals for one and two population means, one and two population proportions, and a population variance.
Skills you'll gain
Details to know

Add to your LinkedIn profile
2 quizzes, 10 assignments
See how employees at top companies are mastering in-demand skills

Build your subject-matter expertise
- Learn new concepts from industry experts
- Gain a foundational understanding of a subject or tool
- Develop job-relevant skills with hands-on projects
- Earn a shareable career certificate

There are 6 modules in this course
Welcome to the course! This module contains logistical information to get you started!
What's included
1 video6 readings1 ungraded lab
In this module you will learn how to estimate parameters from a large population based only on information from a small sample. You will learn about desirable properties that can be used to help you to differentiate between good and bad estimators. We will review the concepts of expectation, variance, and covariance, and you will be introduced to a formal, yet intuitive, method of estimation known as the "method of moments".
What's included
10 videos11 readings1 quiz3 assignments1 programming assignment1 ungraded lab
In this module we will learn what a likelihood function is and the concept of maximum likelihood estimation. We will construct maximum likelihood estimators (MLEs) for one and two parameter examples and functions of parameters using the invariance property of MLEs.
What's included
5 videos5 readings2 assignments1 programming assignment1 ungraded lab
In this module we will explore large sample properties of maximum likelihood estimators including asymptotic unbiasedness and asymptotic normality. We will learn how to compute the “Cramér–Rao lower bound” which gives us a benchmark for the smallest possible variance for an unbiased estimator.
What's included
5 videos5 readings2 assignments1 programming assignment1 ungraded lab
In this module we learn about the theory of “interval estimation”. We will learn the definition and correct interpretation of a confidence interval and how to construct one for the mean of an unseen population based on both large and small samples. We will look at the cases where the variance is known and unknown.
What's included
5 videos5 readings1 quiz1 assignment1 programming assignment2 ungraded labs
In this module, we will generalize the lessons of Module 4 so that we can develop confidence intervals for other quantities of interest beyond the distribution mean and for other distributions entirely. This module covers two sample confidence intervals in more depth, and confidence intervals for population variances and proportions. We will also learn how to develop confidence intervals for parameters of interest in non-normal distributions.
What's included
5 videos5 readings2 assignments1 ungraded lab
Earn a career certificate
Add this credential to your LinkedIn profile, resume, or CV. Share it on social media and in your performance review.
Build toward a degree
This course is part of the following degree program(s) offered by University of Colorado Boulder. If you are admitted and enroll, your completed coursework may count toward your degree learning and your progress can transfer with you.¹
Instructor

Offered by
Explore more from Probability and Statistics
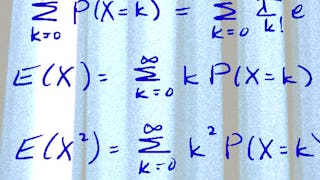 Status: Free Trial
Status: Free TrialUniversity of Colorado Boulder
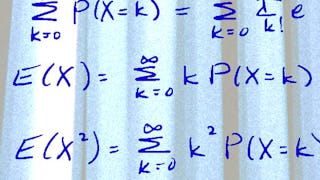 Status: Free Trial
Status: Free TrialUniversity of Colorado Boulder
 Status: Free Trial
Status: Free TrialUniversity of Colorado Boulder
 Status: Free Trial
Status: Free TrialUniversity of Colorado Boulder
Why people choose Coursera for their career




Learner reviews
88 reviews
- 5 stars
57.95%
- 4 stars
18.18%
- 3 stars
6.81%
- 2 stars
6.81%
- 1 star
10.22%
Showing 3 of 88
Reviewed on Jul 18, 2024
This course provided me with truly deep insights into the inner workings of statistics. Thank you very much.
Reviewed on Feb 27, 2024
The overall experience of the course was good but some of the questions were wacky at times
Reviewed on Jan 27, 2024
Excellent. Challenging quizzes that really make you apply the points from the lectures. Very detailed course that has taken me to the next level of my understanding of statistical inference.

Open new doors with Coursera Plus
Unlimited access to 10,000+ world-class courses, hands-on projects, and job-ready certificate programs - all included in your subscription
Advance your career with an online degree
Earn a degree from world-class universities - 100% online
Join over 3,400 global companies that choose Coursera for Business
Upskill your employees to excel in the digital economy
Frequently asked questions
To access the course materials, assignments and to earn a Certificate, you will need to purchase the Certificate experience when you enroll in a course. You can try a Free Trial instead, or apply for Financial Aid. The course may offer 'Full Course, No Certificate' instead. This option lets you see all course materials, submit required assessments, and get a final grade. This also means that you will not be able to purchase a Certificate experience.
When you enroll in the course, you get access to all of the courses in the Specialization, and you earn a certificate when you complete the work. Your electronic Certificate will be added to your Accomplishments page - from there, you can print your Certificate or add it to your LinkedIn profile.
Yes. In select learning programs, you can apply for financial aid or a scholarship if you can’t afford the enrollment fee. If fin aid or scholarship is available for your learning program selection, you’ll find a link to apply on the description page.
More questions
Financial aid available,